
background
There is a variety of stereoscopic phenomena in which the perceived slant or curvature of a surface patch is not what one would predict from the disparities.
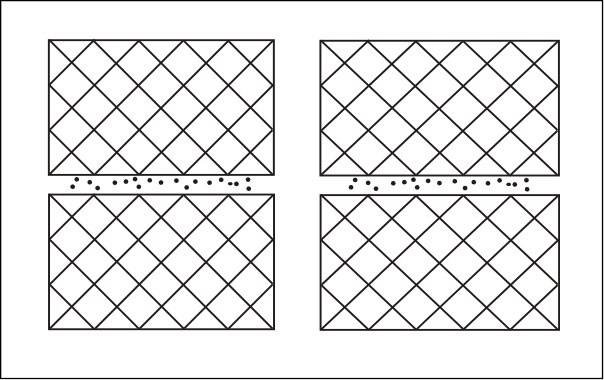
An example is illustrated on the left. Cross your eyes such that the left eye is directed toward the middle of the right image and right eye toward the left image (This is cross-fusing.) You should see three images; we're interested in the middle one. The cross-hatched panels at the top and bottom are wider in the right eye's image than in the left eye's, so they have a disparity gradient specifying that the panels are slanted left side away. The row of dots is identical in the two eyes, so they have a disparity gradient specifying that they are not slanted. Yet you see the row of dots as closer on the left and farther on the right. This is the slant-contrast illusion.
slant contrast experiment
We examined this illusion in a series of experiments and developed a theory for this class of stereoscopic illusion. In the first experiment, we asked people to adjust the disparities in the row of dots (the test strip) until it appeared to have a slant of 0 (which means that it appeared to be in the plane of the computer monitor). People experienced a large and consistent illusion so they had to null the perceived slant by adding a disparity gradient in the same direction as the disparity gradient of the inducing panels above and below the test strip. Those settings are represented by the blue data points below. The figure plots the actual slant of the test strip that appeared to be 0 as a function of the disparity-specified slant of the inducing panels. The three panels correspond to different viewing distances. If no illusion occurred, the data would lie on the black horizontal lines.
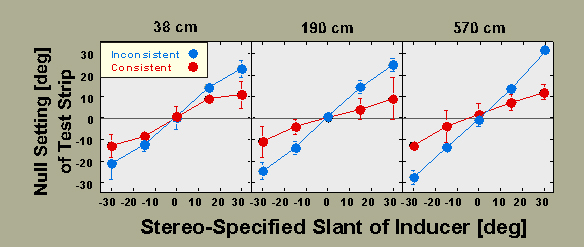
The theory is a Bayesian-like scheme for estimating the slants of the inducing panels and test strip. Specifically, it determines the slants via linear combination of various slant estimators (disparity- and texture-based estimators from the test strip and inducing panel). The weight of each estimator is proportional to the inverse variance of the estimator output. The theory explains slant contrast because the absolute slant of the inducers and the relative slant between the test strip and inducers are both estimated with greater reliability than the absolute slant of the test strip. The theory predicts that slant contrast will be eliminated if the signals specifying the inducers' slant (that is, the disparity and texture gradient signals) are consistent with one another. To test this idea, we made the texture gradient in the inducing panels consistent with the disparity gradient. The red points represent the data when the disparity and texture gradients were consistent with one another. As you can see, the illusion was diminished, but not eliminated. This led us to the following experiment.
When an experiment is performed on a computer display (or any other fixed surface), the visual system always receives signals specifying that the stimulus is in the plane of the display screen. These signals include graininess due to pixelization (the graininess is constant and therefore specifies that the stimulus is not slanted), motion parallax due to motion of the observer's head (the motion parallax is consistent with an unslanted stimulus), and blur (the blur is constant across the screen and is a cue to flatness). We wondered if the residual slant contrast illusion that we observed in the "consistent" condition in the previous experiment could be due to these flatness cues.
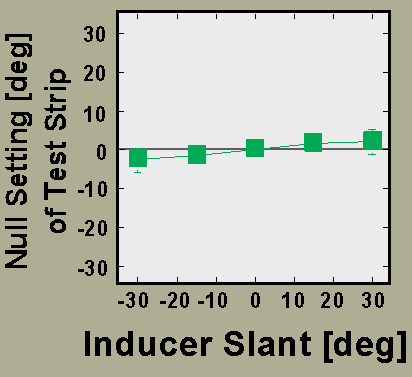
To test this hypothesis, we redid the experiment with a stimulus constructed of real planes. The lighting was done carefully to avoid highlights and shadows. The observers adjusted a panel containing luminous dots until the test strip appeared to have a slant of 0. The data are shown on the left. The slant contrast illusion was eliminated by the use of real surfaces! This means that the conflicting cues provided by presentation on a computer display had produced the illusion in the "consistent" condition in the previous experiment. We are currently investigating how display-screen surface cues affect space perception in a variety of setting.




